Order of operations
The order of operations is a very simple, but a very important rule. So important, in fact, that you will have to obide by it in every math problem you encounter – ever! It concernes the order in which these operations need to be performed. The order of operations lists mathematical operations in order in which they should be performed in a mathematical expression, from highest to lowest priority. Here is that list:
- Parentheses or brackets
- Exponents and roots
- Multiplication and division
- Addition and subtraction
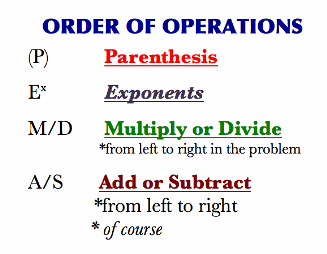
The first letters of these operations (P-E-M-A) are often used as a mnemonic device to help you remember the order in which they should be performed. As you can see, operations inside parentheses have the highest priority, then exponents, then multiplication and, with the lowest priority, addition. To explain how this translates to problem solving, we will use this example:
(8 – 10)2 – (-9) * (-3)
This is a fairly complex example, but it contains all the elements needed to properly explain the order of operations . The first step we have to do is to get rid of the parentheses where it is possible. That can be done by solving the expressions inside the parentheses.
(-2)2 – (-9) * (-3)
Notice that we left -9 and -3 inside their parentheses. That is because there are no operations to perform there and we will get rid of them a bit later. We will leave them be to avoid clutter. Now we need to perform the exponentation, as it is second on the list of priorities. Since (-2)2 equals 4, we get:
4 – (-9) * (-3)
The next operation on the order of operations is multiplication, so let us do it. The result of multiplying (-9) and (-3) is 27, so we are left with:
4 – 27
The only operation left to perform now is subtraction, which has the lowest priority. The end result is:
-23
It is important to adhere to this order as it affects the end result. If we performed the operations in a different order, we could have gotten the wrong result.
If you wish to practice the order of operations, you can use the free worksheets below.
Order of operations exams for teachers
Order of operations worksheets for students
| Worksheet Name | File Size | Downloads | Upload date |
| Order of operations – Positive numeric expressions | 781.9 kB | 7255 | September 3, 2019 |
| Order of operations – Non positive numeric expressions | 843.8 kB | 4916 | September 3, 2019 |
| Order of operations – Positive algebraic expressions | 924.8 kB | 4242 | September 3, 2019 |
| Order of operations – Non positive algebraic expressions | 1.4 MB | 3917 | September 3, 2019 |